Average Interest Rate of Continuously Compounding
Continuously Compounded Interest
Interest that is computed on the initial principal as well as all interest earned by the principal in the past
What is Continuously Compounded Interest?
Continuously compounded interest is interest that is computed on the initial principal, as well as all interest other interest earned. The idea is that the principal will receive interest at all points in time, rather than in a discrete way at certain points in time.

The continuous payment of interest leads to exponential growth and is many times used as an argument for wealth creation. Albert Einstein is credited with the phrase "compound interest is the most powerful force in the universe." While it is undetermined if he actually said it, it says a lot about the importance of the concept.
To understand continuously compounded interest, we will quickly review simple interest and compound interest.
Consider the following example: An investor invests $1,000 in a 5-year term deposit that pays a continuously compounded interest of 6%.
What is Simple Interest?
Simple interest is only computed on the initial principal and not on any interest earned by the initial principal amount. Consider the following example: An investor invests $1,000 in a 5-year term deposit paying a simple interest of 6%.
Total Interest Earned = Principal * Interest * Time
Total Interest Earned = $1,000 * .06 * 5 = $300
Average Annual Interest = Total Interest Earned / Time
Average Annual Interest = $300 / 5 = $60
What is Compound Interest?
Compound interest is computed on the initial principal as well as on the interest earned by the principal over a specified period of time. Consider the following example: An investor invests $1,000 in a 5-year term deposit with an interest rate of 8% with the interest compounded annually.
Therefore, at the end of each year, the interest amount generated in that year is added to the principal amount. It is the new principal amount and the interest for the next year is generated based on the principal amount.
Total Interest Earned = Principal * [(1 + Interest Rate)Time – 1]
Total Interest Earned = $1,000 * [(1 + .06)5 – 1 = $338.23
Average Annual Interest = Total Interest Earned / Time
Average Annual Interest = $338.23 / 5 = $67.65
Formula for Compounded Interest
General compound interest takes into account interest earned over some previous interval of time.
General Compound Interest = Principal * [(1 + Annual Interest Rate/N)N*Time
Where:
- N is the number of times interest is compounded in a year.
Consider the following example: An investor is given the option of investing $1,000 for 5 years in two deposit options.
- Deposit A pays 6% interest with the interest compounded annually.
- Deposit B pays 6% interest with the interest compounded quarterly.
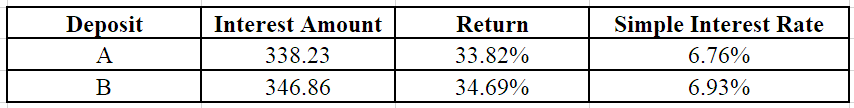
Clearly, Deposit B is a better option as it provides a higher return.
Continuously Compounded Interest Formula
Continuously compounded interest is the mathematical limit of the general compound interest formula, with the interest compounded an infinitely many times each year. Or in other words, you are paid every possible time increment. Mathematicians, have derived a way to approximate the value such a sum would converge to, and it is given by the following formula:

Where:
- N is the number of times interest is compounded in a year.
Continuously compounded interest is the mathematical limit of the general compound interest formula with the interest compounded an infinitely many times each year. Consider the example described below.
- Initial principal amount is $1,000.
- Rate of interest is 6%.
- The deposit is for 5 years.
Total Interest Earned = Principal * [(eInterest Rate*Time) – 1]
Total Interest Earned = $1,000 * [e.06*5 – 1] = $349.86
Average Annual Interest = Total Interest Earned / Time
Average Annual Interest = $349.86 / 5 = $69.97
Table of Interest Payments and Total Return
Consider the example described above.
- Initial principal amount is $1,000.
- Rate of interest is 6%.
- The deposit is for 5 years.
| No. of Compounding Periods Each Year | Interest Amount | Return (in %) |
|---|---|---|
| 1 | 338.2256 | 33.82256 |
| 2 | 343.9164 | 34.39164 |
| 3 | 345.8683 | 34.58683 |
| 4 | 346.855 | 34.6855 |
| 5 | 347.4505 | 34.74505 |
| 6 | 347.8489 | 34.78489 |
| 7 | 348.1342 | 34.81342 |
| 8 | 348.3486 | 34.83486 |
| 9 | 348.5156 | 34.85156 |
| 10 | 348.6493 | 34.86493 |
| 11 | 348.7588 | 34.87588 |
| 12 | 348.8502 | 34.88502 |
| 13 | 348.9275 | 34.89275 |
| 14 | 348.9938 | 34.89938 |
| 15 | 349.0513 | 34.90513 |
| 16 | 349.1016 | 34.91016 |
| 17 | 349.146 | 34.9146 |
| 18 | 349.1855 | 34.91855 |
| 19 | 349.2209 | 34.92209 |
| 20 | 349.2527 | 34.92527 |
| 100 | 349.7374 | 34.97374 |
| 1,000 | 349.8467 | 34.98467 |
| 10,000 | 349.8576 | 34.98576 |
| 100,000 | 349.8587 | 34.98587 |
More Resources
Thank you for reading CFI's guide to Continuously Compounded Interest. To keep learning and developing your knowledge of financial analysis, we highly recommend the additional CFI resources below:
- Math for Corporate Finance Course
- Continuously Compounded Return
- Effective Annual Interest Rate
- Interest Income
Source: https://corporatefinanceinstitute.com/resources/knowledge/finance/continuously-compounded-interest/
0 Response to "Average Interest Rate of Continuously Compounding"
Post a Comment